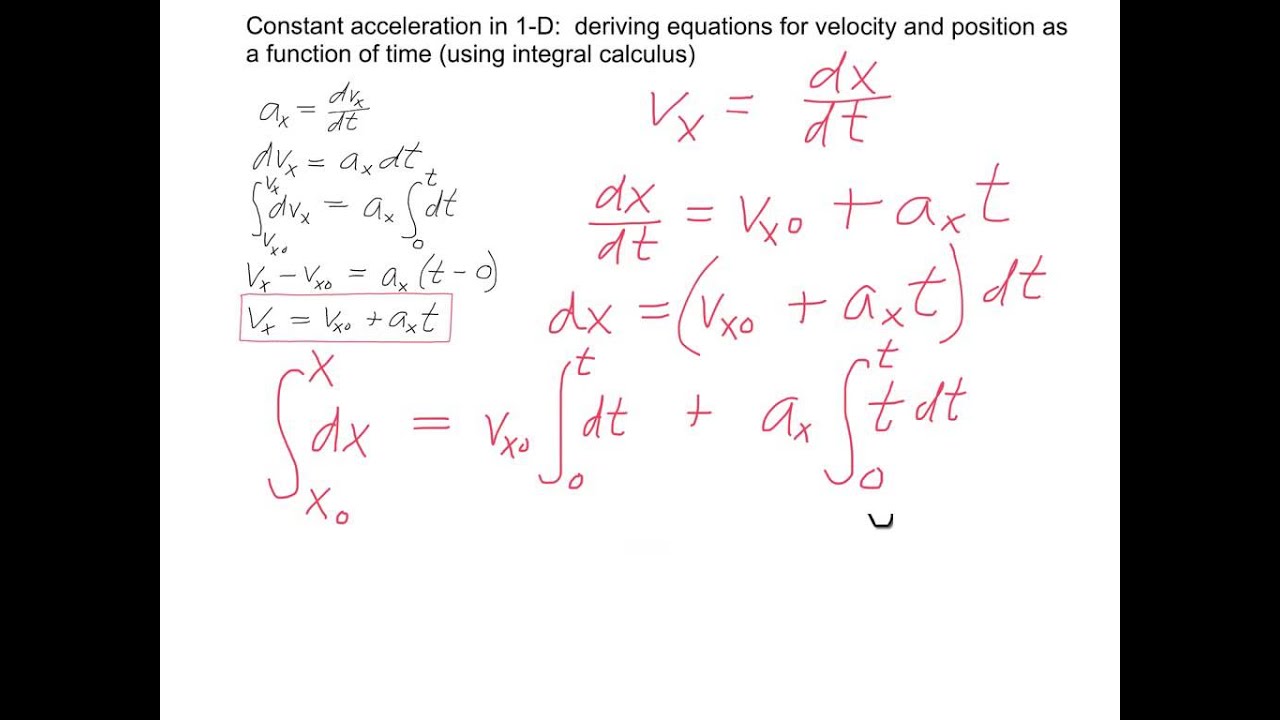
lookimanual.blogg.se - Velocity equation physics

VELOCITY EQUATION PHYSICS PLUS

We can use this knowledge (and our knowledge of integrals) to derive the kinematics equations.įirst, we need to establish that acceleration is represented by the equation a(t) = -9.8.īecause velocity is the antiderivative of acceleration, that means that v'(t) = a(t) and v(t) = int. We know that acceleration is approximately -9.8 m/s^2 (we're just going to use -9.8 so the math is easier) and we know that acceleration is the derivative of velocity, which is the derivative of position. We usually start with acceleration to derive the kinematic equations.

VELOCITY EQUATION PHYSICS PLUS

3 m/s ) 2 − 4 t, equals, start fraction, minus, 18, point, 3, start text, space, m, slash, s, end text, plus minus, square root of, left parenthesis, 18, point, 3, start text, space, m, slash, s, end text, right parenthesis, squared, minus, 4, open bracket, start fraction, 1, divided by, 2, end fraction, left parenthesis, minus, 9, point, 81, start fraction, start text, space, m, end text, divided by, start text, space, s, end text, squared, end fraction, right parenthesis, left parenthesis, minus, 12, point, 2, start text, space, m, end text, right parenthesis, close bracket, end square root, divided by, 2, open bracket, start fraction, 1, divided by, 2, end fraction, left parenthesis, minus, 9, point, 81, start fraction, start text, space, m, end text, divided by, start text, space, s, end text, squared, end fraction, right parenthesis, close bracket, end fraction

Since our H values seem to be getting very close to 7, we can say that 7 meters/second is a good estimate for the instantaneous velocity at (1,3).For instance, say we knew a book on the ground was kicked forward with an initial velocity of v 0 = 5 m/s v_0=5\text t = 2 − 1 8.

Then, it's just a matter of finding our H values and making an estimation. For this problem, we'll use (1,3) as our P point, but we'll have to find a few other points near it to use as our Q points.

Use graphical estimation to find the instantaneous velocity at (1,3) for the displacement equation s = 4t 2 - t. Generally, your graph won't extend behind the y axis - we don't often measure velocity for objects moving backward in time! If the line representing your object's motion drops below the x axis, this represents your object moving behind where it started. Note that the graph can extend below the x axis.Then, just plot points by plugging values for t into your displacement equation, getting s values for your answers, and marking the t,s (x,y) points on the graph. To graph an object's displacement, use the x axis to represent time and the y axis to represent displacement.X Research source In fact, if you represent an object's displacement with a line on a graph, the slope of the line at any given point is equal to the object's instantaneous velocity at that point. In the section above, we mentioned that derivatives are just formulas that let us find the slope at any point for the equation you take the derivative for. Graph your object's displacement over time.